Matematiksel tanıtta mantık kullanılır ancak genellikle bir ölçüde doğal dilden de yararlanılır ve dolayısıyla bir parça belirsizlik içerir. Gerçektende matematikte yazılan tanıtların büyük çoğunluğu informel mantığın uygulaması olarak kabul edilebilir. Tamamıyla formel tanıtların ele alındığı tanıtlama teorisi bağlamında, bu tip tamamıyle formel olmayan tanıtlamalara "sosyal tanıtlama" denir. Bu ayrım, günümüz ve geçmiş matematiksel uygulamaların,matematikte yarı görgücülüğün ve matematik folklorünün yoğun olarak incelenmesine yol açmıştır. Matematik felsefesi ise dilin ve mantığın tanıtlardaki rölü ve "dil olarak matematik" ile ilgilidir.

Kişinin formalizme olan yaklaşımından bağımsız olarak, doğru olduğu tanıtlanan sonucateorem denir. Bu teorem, tamamıyla formel olan bir tanıtta son satırda yer alır ve tanıtın tümü, bu teoremin aksiyomlardan nasıl türetildiğini gösterir. Bir teorem tanıtlandıktan sonra başka önermeleri tanıtlamada kullanılabilir. Matematiğin temelleri adı verilen önermeler tanıtlanamayan ya da tanıtlanması gerekmeyen önermelerdir. Bunlar bir zamanlar matematik felsefecilerinin başlıca uğraşı alanıydı. Günümüzde ilgi odağı daha çokmatematiksel uygulamalara, yani kabul edilebilir matematiksel tekniklere kaymıştır.

Bazı kabul görmüş tanıtlama teknikleri:

• Doğrudan tanıtlama: Sonucun, aksiyomlar, tanımlar ve daha önceki savların mantıksal olarak birleştirilmesiyle elde edildiği yöntem.
• Tümevarımla tanıtlama: Temel bir durumun tanıtlandığı ve bir tümevarım kuralı kulanılarak çok sayıda (sıkça sonsuz olan) başka durumların tanıtlandığı yöntem.
• Olmayana ergi tanıtı (Reductio ad absurdum olarak da bilinir): Bir özelliğin doğru olması durumunda mantıksal bir çelişkinin doğacağı dolayısıyla özelliğin yanlış olduğunun gösterildiği yöntem.
• Oluşturarak tanıtlama: İstenen özelliğe sahip somut bir örnek oluşturularak istenen özellikte bir nesnenin var olduğunun gösterildiği yöntem.
• Tüketerek tanıtlama: Tanıtlanacak önermenin sonlu sayıda duruma bölünerek her birinin ayrı ayrı tanıtlandığı yöntem.

Olasılıkçı tanıtlama, olasılık teorisi yardımıyla istenen özellikte bir örneğin var olduğunun gösterildiği bir tanıtlama olarak anlaşılmalıdır, yani bir teoremin doğru "olabileceği" şeklinde değil. Bu ikinci türdeki uslamlamalara 'usayatkınlık tanıtı' denebilir; Collatz sanısı örneğinde bunun gerçek bir tanıtlamadan ne kadar uzak olduğu aşikardır. Olasılıkçı tanıtlama -oluşturarak tanıtlama dışında- varlık teoremlerini tanıtlamanın birçok yönteminden biridir.

Örneğin "f(X)'i sağlayan en az bir X var" önermesini tanıtlamaya çalışıyorsanız, bir varlık ya da oluşturmacı olmayan tanıt f(X)'i sağlayan bir X olduğunu tanıtlar fakat bu X'in nasıl elde edileceğini göstermez. Buna karşın oluşturmacı bir kanıt X'in nasıl elde edildiğini de gösterir.

Doğru olduğu düşünülen fakat henüz tanıtlanmayan bir önerme sanı (konjektür) olarak bilinir.

Bazı durumlarda, belirli bir önermenin verili bir aksiyomlar kümesinden tanıtlanamayacağı tanıtlanabilir; bkz. örneğin süreklilik hipotezi. Aksiyom sistemlerinin çoğunda, ne tanıtlanabilen ne de tanıtlanamayan önermeler bulunur (bkz. Gödel'in eksiklik kuramı).

In mathematics, a proof is a convincing demonstration (within the accepted standards of the field) that some mathematical statement is necessarily true. Proofs are obtained fromdeductive reasoning, rather than from inductive or empirical arguments. That is, a proof must demonstrate that a statement is true in all cases, without a single exception. An unproved proposition that is believed to be true is known as a conjecture.

The statement that is proved is often called a theorem. Once a theorem is proved, it can be used as the basis to prove further statements. A theorem may also be referred to as alemma, especially if it is intended for use as a stepping stone in the proof of another theorem.

Proofs employ logic but usually include some amount of natural language which usually admits some ambiguity. In fact, the vast majority of proofs in written mathematics can be considered as applications of rigorous informal logic. Purely formal proofs, written in symbolic language instead of natural language, are considered in proof theory. The distinction between formal and informal proofs has led to much examination of current and historical mathematical practice, quasi-empiricism in mathematics, and so-called folk mathematics (in both senses of that term). The philosophy of mathematics is concerned with the role of language and logic in proofs, and mathematics as a language. 

History and etymology

Plausibility arguments using heuristic devices such as pictures and analogies preceded strict mathematical proof..[1] The early history of the concept of proof dates back to the early Greek and Chinese civilisations. Thales (640–546 BCE) proved some theorems in geometry. Eudoxus (408–355 BCE) and Theaetetus (417–369 BCE) formulated theorems but did not prove them. Aristotle (384–322 BCE) said definitions should describe the concept being defined in terms of other concepts already known. Euclid (300 BCE) began with undefined terms and axioms (propositions regarding the undefined terms assumed to be self-evidently true, from the Greek “axios” meaning “something worthy”) and used these to prove theorems using deductive logic. Modern proof theory treats proofs as inductively defined data structures. There is no longer an assumption that axioms are "true" in any sense; this allows for parallel mathematical theories built on alternate sets of axioms (seeAxiomatic set theory and Non-Euclidean geometry for examples).

The word Proof comes from the Latin probare meaning "to test". Related modern words are the English "probe", "proboscis”, "probation", and "probability", and the Spanish "probar" (to smell or taste, or (lesser use) touch or test). The early use of "probity" was in the presentation of legal evidence. A person of authority, such as a nobleman, was said to have probity, whereby the evidence was by his relative authority, which outweighed empirical testimony.[2]

Nature and purpose

There are two different conceptions of mathematical proof.[3] The first is an informal proof, a rigorous natural-language expression that is intended to convince the audience of the truth of a theorem. Because of their use of natural language, the standards of rigor for informal proofs will depend on the audience of the proof. In order to be considered a proof, however, the argument must be rigorous enough; a vague or incomplete argument is not a proof. Informal proofs are the type of proof typically encountered in published mathematics. They are sometimes called "formal proofs" because of their rigor, but logicians use the term "formal proof" to refer to a different type of proof entirely.

In logic, a formal proof is not written in a natural language, but instead uses a formal language consisting of certain strings of symbols from a fixed alphabet. This allows the definition of a formal proof to be precisely specified without any ambiguity. The field of proof theory studies formal proofs and their properties. Although each informal proof can, in theory, be converted into a formal proof, this is rarely done in practice. The study of formal proofs is used to determine properties of provability in general, and to show that certainundecidable statements are not provable.

A classic question in philosophy asks whether mathematical proofs are analytic orsynthetic. Kant, who introduced the analytic-synthetic distinction, believed mathematical proofs are synthetic.

Proofs may be viewed as aesthetic objects, admired for their mathematical beauty. The mathematician Paul Erdős was known for describing proofs he found particularly elegant as coming from "The Book", a hypothetical tome containing the most beautiful method(s) of proving each theorem. The book Proofs from THE BOOK, published in 2003, is devoted to presenting 32 proofs its editors find particularly pleasing.

Methods of proofDirect proof

In direct proof, the conclusion is established by logically combining the axioms, definitions, and earlier theorems. For example, direct proof can be used to establish that the sum of two even integers is always even:

For any two even integers x and y we can write x = 2a and y = 2b for some integers a and b, since both x and y are multiples of 2. But the sum x + y = 2a + 2b = 2(a + b) is also a multiple of 2, so it is therefore even by definition.

This proof uses definition of even integers, as well as distribution law.

 

 Proof by mathematical induction

In proof by mathematical induction, first a "base case" is proved, and then an "induction rule" is used to prove a (often infinite) series of other cases. Since the base case is true, the infinity of other cases must also be true, even if all of them cannot be proved directly because of their infinite number. A subset of induction is infinite descent. Infinite descent can be used to prove the irrationality of the square root of two.

The principle of mathematical induction states that: Let N = { 1, 2, 3, 4, ... } be the set of natural numbers and P(n) be a mathematical statement involving the natural number n belonging to N such that

• (i) P(1) is true, i.e., P(n) is true for n = 1
• (ii) P(n + 1) is true whenever P(n) is true, i.e., P(n) is true implies that P(n + 1) is true.

Then P(n) is true for all natural numbers n.

Mathematicians often use the term "proof by induction" as shorthand for a proof by mathematical induction.[4] However, the term "proof by induction" may also be used in logic to mean an argument that uses inductive reasoning.

 

 Proof by transposition

Proof by transposition establishes the conclusion "if p then q" by proving the equivalentcontrapositive statement "if not q then not p".

 

Proof by contradiction

In proof by contradiction (also known as reductio ad absurdum, Latin for "by reduction toward the absurd"), it is shown that if some statement were so, a logical contradiction occurs, hence the statement must be not so. This method is perhaps the most prevalent of mathematical proofs. A famous example of a proof by contradiction shows that 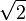 is anIrrational number:

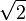
Suppose that  is a rational number, so  where a and b are non-zero integers with no common factor (definition of a rational number). Thus, . Squaring both sides yields 2b2 = a2. Since 2 divides the left hand side, 2 must also divide the right hand side (as they are equal and both integers). So a2 is even, which implies that a must also be even. So we can write a = 2c, where c is also an integer. Substitution into the original equation yields 2b2 = (2c)2 = 4c2. Dividing both sides by 2 yields b2 = 2c2. But then, by the same argument as before, 2 divides b2, so b must be even. However, if a and b are both even, they share a factor, namely 2. 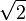This contradicts our assumption, so we are forced to conclude that  is an irrational number.

 

Proof by construction

Proof by construction, or proof by example, is the construction of a concrete example with a property to show that something having that property exists. Joseph Liouville, for instance, proved the existence of transcendental numbers by constructing an explicit example.

 

Proof by exhaustion

In proof by exhaustion, the conclusion is established by dividing it into a finite number of cases and proving each one separately. The number of cases sometimes can become very large. For example, the first proof of the four colour theorem was a proof by exhaustion with 1,936 cases. This proof was controversial because the majority of the cases were checked by a computer program, not by hand. The shortest known proof of the four colour theorem today still has over 600 cases.

 

Probabilistic proof

A probabilistic proof is one in which an example is shown to exist, with certainty, by using methods of probability theory. This is not to be confused with an argument that a theorem is 'probably' true. The latter type of reasoning can be called a 'plausibility argument' and is not a proof; in the case of the Collatz conjecture it is clear how far that is from a genuine proof.[5] Probabilistic proof, like proof by construction, is one of many ways to show existence theorems.

 

Combinatorial proof

A combinatorial proof establishes the equivalence of different expressions by showing that they count the same object in different ways. Often a bijection between two sets is used to show that the expressions for their two sizes are equal. Alternatively, a double counting argument provides two different expressions for the size of a single set, again showing that the two expressions are equal.

 

Nonconstructive proof

A nonconstructive proof establishes that a certain mathematical object must exist (e.g. "Some X satisfies f(X)"), without explaining how such an object can be found. Often, this takes the form of a proof by contradiction in which the nonexistence of the object is proven to be impossible. In contrast, a constructive proof establishes that a particular object exists by providing a method of finding it. A famous example of a nonconstructive proof shows that there exist two irrational numbers a and b such that ab is a rational number:

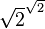
Either  is a rational number and we are done (take ), 
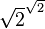
or  is irrational so we can write  and . 
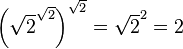
This then gives , which is thus a rational 
of the form ab.

 

Visual proof

Although not a formal proof, a visual demonstration of a mathematical theorem is sometimes called a "proof without words". The picture at right is an example of a historic visual proof of the Pythagorean theorem in the case of the (3,4,5) triangle.

 

Elementary proof

An elementary proof is a proof which only uses basic techniques. More specifically, the term is used in number theory to refer to proofs that make no use of complex analysis. For some time it was thought that certain theorems, like the prime number theorem, could only be proved using "higher" mathematics. However, over time, many of these results have been reproved using only elementary techniques.

 

Two-column proof

A particular form of proof using two parallel columns is often used in elementary geometry classes.[6] The proof is written as a series of lines in two columns. In each line, the left hand column contains propositions (or sometimes called statements), while the right hand column contains a brief explanation of how this proposition is either an axiom, a hypothesis, or can be obtained from previous lines (or sometimes just called "reasons").

 

Statistical proofs in pure mathematics

The expression "statistical proof" may be used technically or colloquially in areas of pure mathematics, such as involving cryptography, chaotic series, and probabilistic or analyticnumber theory.[7][8][9] It is less commonly used to refer to a mathematical proof in the branch of mathematics known as mathematical statistics. See also "Statistical proof using data" section below.[4].

 

Computer-assisted proofs

Until the twentieth century it was assumed that any proof could, in principle, be checked by a competent mathematician to confirm its validity.[1] However, computers are now used both to prove theorems and to carry out calculations that are too long for any human or team of humans to check; the first proof of the four color theorem is an example of a computer-assisted proof. Some mathematicians are concerned that the possibility of an error in a computer program or a run-time error in its calculations calls the validity of such computer-assisted proofs into question. In practice, the chances of an error invalidating a computer-assisted proof can be reduced by incorporating redundancy and self-checks into calculations, and by developing multiple independent approaches and programs.

 

Undecidable statements

A statement that is neither provable nor disprovable from a set of axioms is called undecidable (from those axioms). One example is the parallel postulate, which is neither provable nor refutable from the remaining axioms of Euclidean geometry.

Mathematicians have shown there are many statements that are neither provable nor disprovable in Zermelo-Fraenkel set theory with the axiom of choice (ZFC), the standard system of set theory in mathematics (assuming that ZFC is consistent); see list of statements undecidable in ZFC.

Gödel's (first) incompleteness theorem shows that many axiom systems of mathematical interest will have undecidable statements.

 

Heuristic mathematics 
and experimental mathematics

While early mathematicians such as Eudoxus of Cnidus did not use proofs, from Euclid to the foundational mathematics developments of the late 19th and 20th centuries, proofs were an essential part of mathematics.[10] With the increase in computing power in the 1960’s, significant work began to be done investigating mathematical objects outside of the proof-theorem framework,[11] in experimental mathematics. Early pioneers of these methods intended the work ultimately to be embedded in a classical proof-theorem framework, e.g. the early development of fractal geometry[12], which was ultimately so embedded.

 

 Related concepts

 

Colloquial use of "mathematical proof"

The expression "mathematical proof" is used by lay people to refer to using mathematical methods or arguing with mathematical objects, such as numbers, to demonstrate something about everyday life, or when data used in an argument are numbers. It is sometime also used to mean a "statistical proof" (below), especially when used to argue from data.

 

Statistical proof using data

"Statistical proof" from data refers to the application of statistics, data analysis, orBayesian analysis to infer propositions regarding the probability of data. While using mathematical proof to establish theorems in statistics, it is usually not a mathematical proof in that the assumpions from which probability statements are derived require empirical evidence from outside mathematics to verify. In physics, in addition to statistical methods, "statistical proof" can refer to the specialized mathematical methods of physicsapplied to analyze data in a particle physics experiment or observational study incosmology. "Statistical proof" may also refer to raw data or a convincing diagram involving data, such as scatter plots, when the data or diagram is adequately convincing without further anaylisis.

 

Inductive logic proofs and Bayesian analysis

Proofs using inductive logic, while considered mathematical in nature, seek to establish propositions with a degree of certainty, which acts in a similar manner to probability, and may be less than one certainty. Bayesian analysis establishes assertions as to the degree of a person's subjective belief. Inductive logic should not be confused with mathematical induction.

 

Proofs as mental objects

Psychologism views mathematical proofs as psychological or mental objects. Mathematician philosophers, such as Leibnitz, Frege, and Carnap, have attempted to develop a semantics for what they considered to be the language of thought, whereby standards of mathematical proof might be applied to empirical science.

 

 Influence of mathematical proof methods outside mathematics

Philosopher-mathematicians such as Schopenhauer have attempted to formulate philosophical arguments in an axiomatic manner, whereby mathematical proof standards could be applied to argumentation in general philosophy. Other mathematician-philosophers have tried to use standards of mathematical proof and reason, without empiricism, to arrive at statements outside of mathematics, but having the certainty of propositions deduced in a mathematical proof, such as Descarte’s cogito argument. Kantand Frege considered mathematical proof to be analytic apriori.